
Īpplying a similar argument, for consistency with the index laws, we define a = , Note that we have defined a to be the positive square root of We do this so that there is only one value for a.

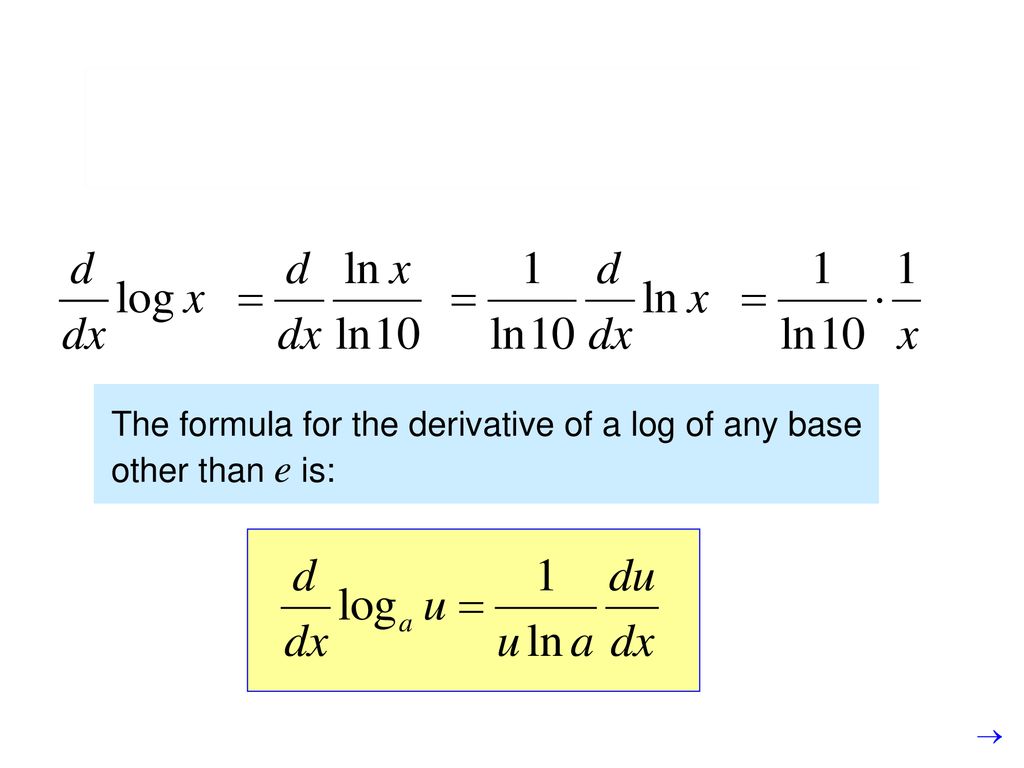
In general we define a = for any positive number a. Hence, squaring this expression we would like to say: Once again, we would like the established index laws to hold. In particular, can we give meaning to 4 ? We now extend our study of indices to include rational or fractional exponents. In each case the form of the terms approaches 0 0.Ī similar situation occurs with and so the expression is also often referred to as an indeterminant form.įor all intergers m and n and non-zero numbers a and b the following are true. (The explanation of this term is that one can find sequences of numbers of the form a b in which both a and b approach 0, but where the limit of the sequence is not 1 and indeed can be made to be any number we like, by a suitable choice of and For example, the terms of the sequenceĪre all equal to 1, while the terms of the sequenceĪre all equal to 0. It is sometimes called an indeterminant form. More generally, if a ≠ 0 then we define a 0 = 1. If the index laws are to be applied in this situation, then we need to define 5 0 to be 1. On the other hand, applying index law 2, ignoring the condition m > n, The basic principle we use throughout is to choose a meaning that is consistent with the index laws above.Ĭlearly = 1. We now seek to give meaning to other types of exponents. Such functions are used to model growth rates in biology, ecology and economics, as well as radioactive decay in nuclear physics. The function in calculus that is a multiple of its own derivative is an exponential function. Thus, to differentiate or integrate a function such as, it is first necessary to convert it to index form. In senior mathematics, competency in manipulating indices is essential, since they are used extensively in both differential and integral calculus. Logarithms can be used to solve equations such as 2 x = 3, for x. This is a very useful tool in experimental science. When two measured quantities appear to be related by an exponential function, the parameters of the function can be estimated using log plots. Is also defined using the notion of a logarithm. the pH value in chemistry, that is used to define the level of acidity of a substance,.the Richter scale, that is used to measure earthquake intensity, is defined using logarithms.decibels, that are used to measure sound pressure, are defined using logarithms.This leads to the notion of a logarithm, which is simply another name for an index. We can reverse this question and ask, for example, ‘What power of 2 gives 16? Our attention is then turned to the index itself. In many applications of mathematics, we can express numbers as powers of some given base. These questions will be considered in this module.


Indices provide a compact algebraic notation for repeated multiplication.


 0 kommentar(er)
0 kommentar(er)
